Fourier ptychographic microscopy

Introduction

Fourier ptychographic microscopy (FPM) is a novel computational coherent imaging technique for high space-bandwidth product imaging. Mathematically, Fourier ptychographic (FP) reconstruction can be implemented as a phase retrieval optimization process, in which we only obtain low resolution intensity images corresponding to the sub-bands of the sample’s high resolution (HR) spatial spectrum, and aim to retrieve the complex HR spectrum.

Highlights

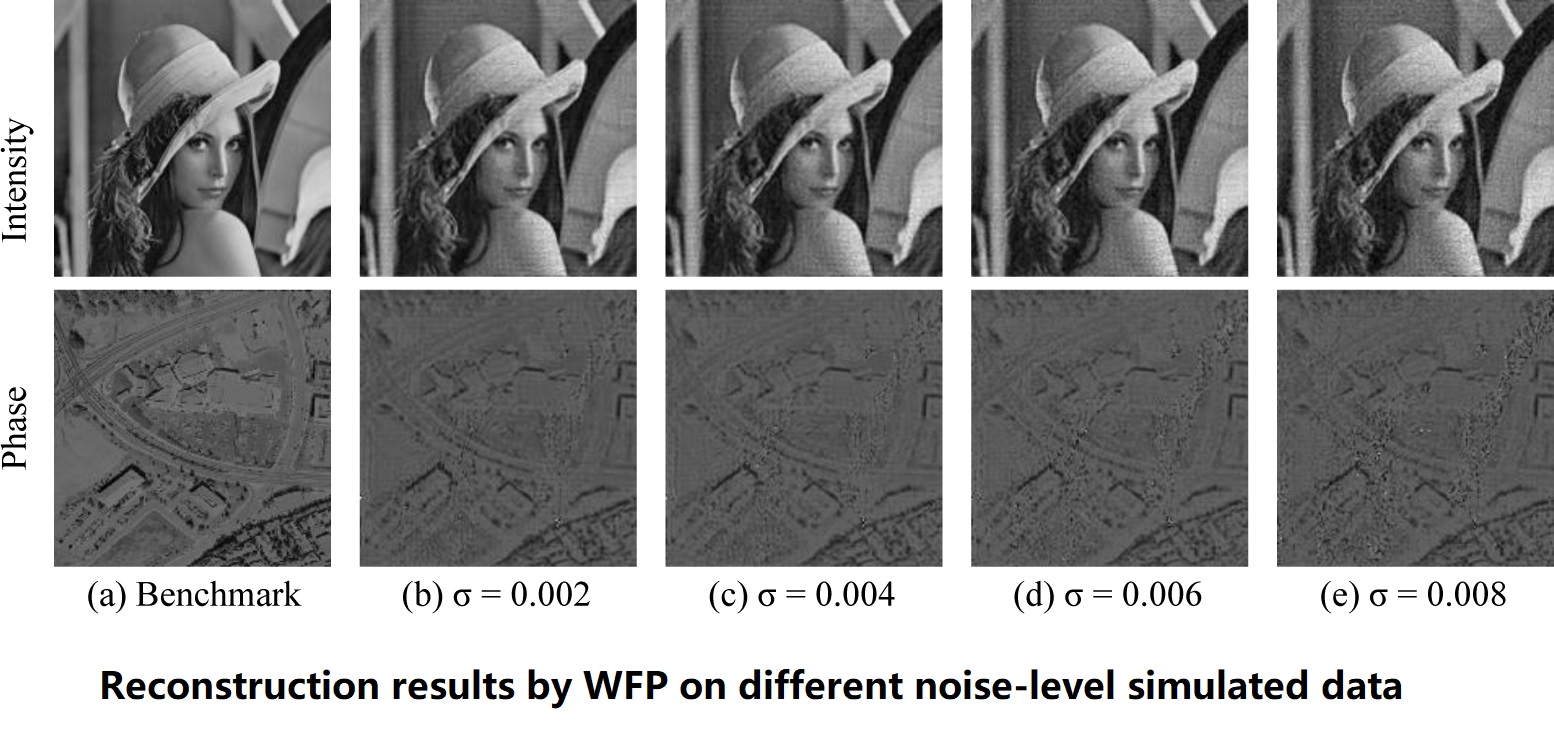
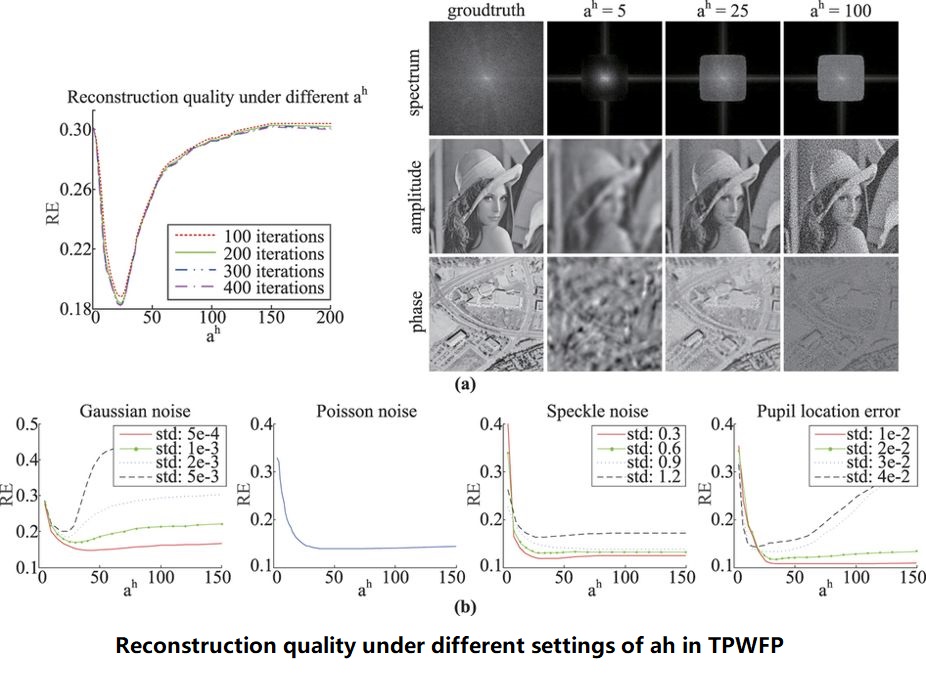
Fourier ptychographic reconstruction using Poisson maximum likelihood and truncated Wirtinger gradient

In real setups, the measurements always suffer from various degenerations such as Gaussian noise, Poisson noise, speckle noise and pupil location error, which would largely degrade the reconstruction. To efficiently address these degenerations, we propose a novel FP reconstruction method under a gradient descent optimization framework in this paper. The technique utilizes Poisson maximum likelihood for better signal modeling, and truncated Wirtinger gradient for effective error removal. Results on both simulated data and real data captured using our laser-illuminated FPM setup show that the proposed method outperforms other state-of-the-art algorithms. Also, we have released our source code for non-commercial use.

Bian, L., Suo, J., Chung, J., Ou, X., Yang, C., Chen, F., & Dai, Q. (2016). Fourier ptychographic reconstruction using Poisson maximum likelihood and truncated Wirtinger gradient. Scientific reports, 6, 27384.

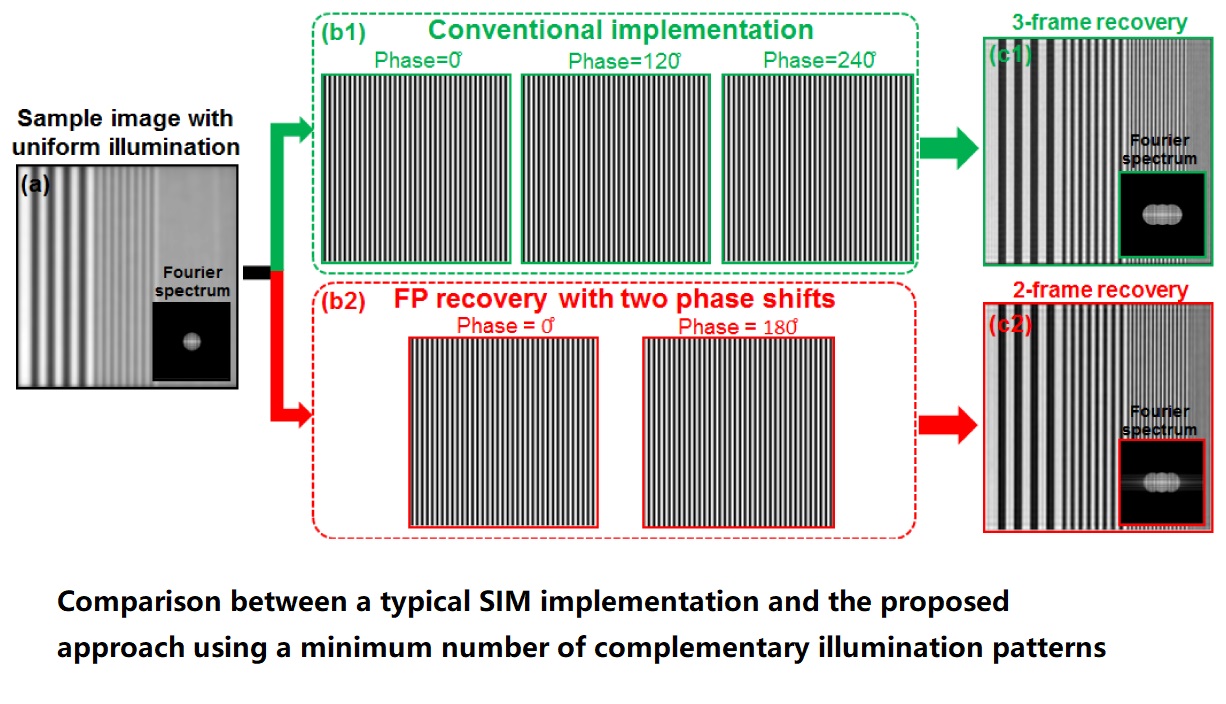
Resolution doubling with a reduced number of image acquisitions

In this paper, we discuss an imaging strategy for the structured illumination technique and demonstrate the use of a modified incoherent Fourier ptychographic procedure for reducing the number of acquisitions. In the first implementation, we used complementary sinusoidal patterns for sample illumination. We show that, the number of lateral phase shifts can be reduced from 3 to 2 for each orientation of the sinusoidal pattern and the total number of image acquisitions can be reduced to 6 with 3 orientations. In the second implementation, we further reduce the number of image acquisitions to 4. We also show that, the resolution-doubled image can be recovered even with unknown phases of the sinusoidal patterns. We validate the proposed imaging procedure with non-fluorescence samples. The reported approach may shorten the acquisition time of super-resolution imaging and reduce phototoxicity of biological samples.

Dong, S., Liao, J., Guo, K., Bian, L., Suo, J., & Zheng, G. (2015). Resolution doubling with a reduced number of image acquisitions. Biomedical optics express, 6(8), 2946-2952.

Content adaptive sparse illumination for Fourier ptychography

In this letter, utilizing the sparsity of natural images in Fourier domain, we propose a highly effcient method termed as AFP, which applies content adaptive sparse illumination for Fourier ptychography by capturing the most informative parts of the scene’s spatial spectrum. We validate the e ectiveness and e ciency of the reported framework with both simulations and real experiments. Results show that the proposed AFP could shorten the acquisition time of conventional FP by around 30%-60%.

Bian, L., Suo, J., Situ, G., Zheng, G., Chen, F., & Dai, Q. (2014). Content adaptive illumination for Fourier ptychography. Optics letters, 39(23), 6648-6651.

Fourier ptychographic reconstruction using Wirtinger flow optimization

In this paper, based on the recently reported Wirtinger flow algorithm, we propose an iterative optimization framework incorporating phase retrieval and noise relaxation together, to realize FP reconstruction using low SNR images captured under short exposure time. Experiments on both synthetic and real captured data validate the effectiveness of the proposed reconstruction method. Specifically, the proposed technique could save   80% exposure time to achieve similar retrieval accuracy compared to the conventional FP. Besides, we have released our source code for non-commercial use.

Bian, L., Suo, J., Zheng, G., Guo, K., Chen, F., & Dai, Q. (2015). Fourier ptychographic reconstruction using Wirtinger flow optimization. Optics express, 23(4), 4856-4866.